Which Of The Following Functions Illustrates A Change In Amplitude

ii.one: Graphs of the Cosine and Sine Functions

Final updated
Salvage as PDF

Page ID: 7104

$Sundstrom.jpg$
Professors (Mathematics) at Grand Valley Country University
Publisher/Source: ScholarWorks @Thousand Valley State University

Focus Questions

The following questions are meant to guide our study of the material in this section. After studying this department, nosotros should understand the concepts motivated by these questions and be able to write precise, coherent answers to these questions.

What are the graphs and important properties of the graphs of $y = \cos(x)$ and $y = \sin(10)$?
What are the domains of the sine and cosine functions? What are the ranges of the sine and cosine functions?
What are the periods of the sine and cosine functions? What does period mean?
What is amplitude? How does the aamplitude affect the graph of the sine or cosine?

Beginning Activity

The nigh basic grade of drawing the graph of a part is to plot points. Utilise the values in the given table to plot the points on the graph of $y = \sin(x)$ so draw the graph of $y = \sin(t)$ for $0 < t < 2\pi$. Note: On the $t$-axis, the gridlines are $\dfrac{\pi}{12}$ units apart and on the $y$-axis, the gridlines are 0.1 of a unit apart.

$imageedit_7_7926200464.png$

$t$	$\sin(t)$	$\sin(t)$ (approx)
0	0	0
$\dfrac{\pi}{6}$	$\dfrac{one}{2}$	0.5
$\dfrac{\pi}{4}$	$\dfrac{\sqrt{2}}{ii}$	0.707
$\dfrac{\pi}{3}$	$\dfrac{\sqrt{3}}{2}$	0.866
$\dfrac{\pi}{2}$	1	ane
$\dfrac{two\pi}{iii}$	$\dfrac{\sqrt{3}}{two}$	0.866
$\dfrac{iii\pi}{4}$	$\dfrac{\sqrt{2}}{two}$	0.714
$\dfrac{five\pi}{vi}$	$\dfrac{ane}{2}$	0.5
$\pi$	0	0
$\dfrac{7\pi}{half-dozen}$	$-\dfrac{1}{ii}$	-0.five
$\dfrac{5\pi}{iv}$	$-\dfrac{\sqrt{ii}}{2}$	-0.707
$\dfrac{4\pi}{3}$	$-\dfrac{\sqrt{3}}{2}$	-0.866
$\dfrac{3\pi}{2}$	-one	-1
$\dfrac{five\pi}{3}$	$-\dfrac{\sqrt{three}}{2}$	-0.866
$\dfrac{vii\pi}{4}$	$-\dfrac{\sqrt{2}}{2}$	-0.707
$\dfrac{eleven\pi}{vi}$	$-\dfrac{one}{ii}$	-0.v
$2\pi$	0	0

Nosotros tin also use a graphing calculator or other graphing device to draw the graph of the sine function. Make certain the device is set to radian mode and apply it to draw the graph of $y = \sin(t)$ using $-2\pi \leq t \leq 4\pi$ and $-1.two \leq t \leq 1.2$. Note: Many graphing utilities crave the use of $x$ every bit the independent variable. For such devices, nosotros demand to utilize $y = \sin(x)$. This will make no difference in the graph of the function.

Compare this to the graph from part (1). What are the similarities? What are the differences?
Discover iv separate values of $t$ where the graph of the sine function crosses the $t$-centrality. Such values are chosen $t$-intercepts of the sine part (or roots or zeros).
Based on the graphs, what appears to be the maximum value of $\sin(t)$. Determine two different values of $t$ that give this maximum value of $\sin(t)$.
Based on the graphs, what appears to be the minimum value of $\sin(t)$. Determine two different values of $t$ that give this minimum value of $\sin(t)$.

The Periods of the Sine and Cosine Functions

1 thing we tin detect from the graphs of the sine function in the start action is that the graph seems to have a "wave" form and that this "moving ridge" repeats as we move forth the horizontal axis. Nosotros encounter that the portion of the graph betwixt 0 and $2\pi$ seems identical to the portion of the graph between $ii\pi$ and $4\pi$ and to the portion of the graph between $-2\pi$ and 0. The graph of the sine role is exhibiting what is known every bit a periodic belongings. Figure two.1 shows the graph of $y = \sin(t)$ for three cycles.

$2.1.png$

Effigy $\PageIndex{1}$: Graph of $y = \sin(t)$ with $-ii\pi \leq t \leq four\pi$

Nosotros say that the sine office is a periodic part. Such functions are often used to model repetitious phenomena such equally a pendulum swinging dorsum and forth, a weight fastened to a spring, and a vibrating guitar string.

The reason that the graph of $y = \sin(t)$ repeats is that the value of $\sin(t)$ is the y-coordinate of a point as it moves around the unit of measurement circle. Since the circumference of the unit circle is $ii\pi$ units, an arc of length $(t + 2\pi)$ will have the same terminal betoken as an arc of length t. Since $\sin(t)$ is the y-coordinate of this point, we see that $\sin(t + ii\pi) = \sin(t)$. This means that the period of the sine function is $2\pi$. Post-obit is a more than formal definition of a periodic part.

Definition

Definition. A function $f$ is periodic with menses $p$ if $f(t + p) = f(t)$ for all $t$ in the domain of $f$ and $p$ is the smallest positive number that has this property.

Notice that if $f$ is a periodic part with menstruation $p$, then if we add 2$p$ to $t$, nosotros get \[f(t + 2p) = f((t+p)+p) = f(t + p) =f(t).\]

We can keep to repeat this process and see that for any integer $thou$, \[f(t + kp) =f(t).\]

And then far, we accept been discussing only the sine part, but nosotros get similar behavior with the cosine role. Recall that the wrapping part wraps the number line around the unit of measurement circumvolve in a mode that repeats in segments of length $2\pi$. This is periodic behavior and information technology leads to periodic beliefs of both the sine and cosine functions since the value of the sine part is the $y$-coordinate of a point on the unit circle and the value of the cosine function is the $x$-coordinate of the same betoken on the unit circumvolve, the sine and cosine functions echo every time we brand i wrap around the unit of measurement circle. That is, \[\cos(t + 2\pi) = \cos(t) \space and \infinite \sin(t + 2\pi) = \sin(t).\]
It is important to recognize that $two\pi$ is the smallest number that makes this happen. Therefore, the cosine and sine functions are periodic with period $two\pi$.

Practice $\PageIndex{1}$

We can, of course, use a graphing utility to draw the graph of the cosine function. However, it does assistance to empathise the graph if we actually draw the graph by paw every bit we did for the sine role in the kickoff activity. Utilize the values in the given table to plot the points on the graph of $y = \cos(t)$ and and then depict the graph of $y = \cos(t)$ for $0 \leq t \leq ii\pi$.

$imageedit_7_7926200464.png$

$t$	$\cos(t)$	$\cos(t)$ (approx)
$0$	$1$	$1$
$\dfrac{\pi}{half-dozen}$	$\dfrac{\sqrt{three}}{2}$	$0.866$
$\dfrac{\pi}{four}$	$\dfrac{\sqrt{2}}{ii}$	$0.707$
$\dfrac{\pi}{3}$	$\dfrac{1}{two}$	$0.5$
$\dfrac{\pi}{2}$	$0$	$0$
$\dfrac{2\pi}{3}$	$-\dfrac{one}{two}$	$-0.5$
$\dfrac{three\pi}{iv}$	$-\dfrac{\sqrt{2}}{2}$	$-0.714$
$\dfrac{5\pi}{6}$	$-\dfrac{\sqrt{three}}{2}$	$-0.866$
$\pi$	$-1$	$-1$
$\dfrac{7\pi}{half-dozen}$	$-\dfrac{\sqrt{3}}{2}$	$-0.866$
$\dfrac{5\pi}{iv}$	$-\dfrac{\sqrt{ii}}{2}$	$-0.714$
$\dfrac{4\pi}{3}$	$-\dfrac{1}{two}$	$-0.5$
$\dfrac{three\pi}{2}$	$0$	$-one$
$\dfrac{5\pi}{iii}$	$\dfrac{i}{2}$	$0.5$
$\dfrac{7\pi}{iv}$	$-\dfrac{\sqrt{2}}{2}$	$0.707$
$\dfrac{11\pi}{half-dozen}$	$\dfrac{\sqrt{3}}{ii}$	$-0.866$
$2\pi$	$one$	$i$

Answer

Non all of the points are plotted, but the following is a graph of one complete catamenia of $y = \cos(t)$ for $0 \leq t \leq 2\pi$.

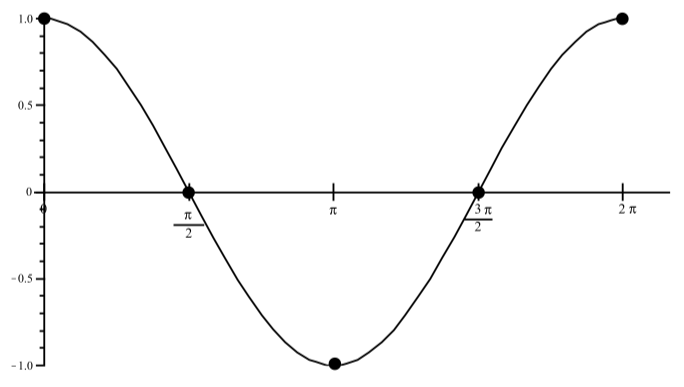
$pc 2.1.png$

$2.2.png$

Figure $\PageIndex{2}$: Graph of $y = \cos(t)$ with $-2\pi \leq t \leq iv\pi$

Do $\PageIndex{2}$

Compare the graph in Effigy $\PageIndex{2}$ to the graph from Practise $\PageIndex{1}$. What are the similarities? What are the differences?
Find four carve up values of $t$ where the graph of the cosine function crosses the $t$-centrality. Such values are chosen $t$-intercepts of the cosine office (or roots or zeros).
Based on the graphs, what appears to be the maximum value of $\cos(t)$. Make up one's mind two dissimilar values of $t$ that give this maximum value of $\cos(t)$.
Based on the graphs, what appears to be the minimum value of $\cos(t)$. Make up one's mind two unlike values of $t$ that give this minimum value of $\cos(t)$.

Respond

The departure is that the graph in Figure $\PageIndex{2}$ shows three complete periods of $y = \cos(t)$ over the interval $[-two\pi, four\pi]$.
The graph of $y = \cos(t)$ has $t$-intercepts at $t = -\dfrac{3\pi}{2}, t = -\dfrac{\pi}{ii}, t = \dfrac{\pi}{2}, t = \dfrac{three\pi}{2}, t = \dfrac{v\pi}{2}$, and $t = \dfrac{7\pi}{ii}$
The maximum value of $y = \cos(t)$ is $ane$. The graph attains this maximum at $t = -2\pi, t = 0, t = two\pi$, and $t = 4\pi$.
The minimum value of $y = \cos(t)$ is $-1$. The graph attains this minimum at $t = -\pi, t = \pi$, and $t = 3\pi$

Activity 2.3 (The Graphs of the Sine and Cosine Functions).

We take now synthetic the graph of the sine and cosine functions by plotting points and past using a graphing utility. Nosotros can accept a improve understanding of these graphs if nosotros tin see how these graphs are related to the unit circle definitions of $\sin(t)$ and $\cos(t)$. We will use 2 Geogebra applets to help united states of america do this.

The first applet is called Sine Graph Generator. The web address is gvsu.edu/due south/Ly

To begin, but move the slider for $t$ until you get $t = ane$ and find the resulting image. On the left, there will be a copy of the unit circle with an arc drawn that has length $1$. The $y$-coordinate of the final point of this arc ($0.84$ rounded to the nearest hundredth) will also be displayed. The horizontal line will exist connected to the point $(1, 0.84)$ on the graph of $y = \sin(t)$. As the values of $t$ are changed with the slider, more points volition be drawn in this mode on the graph of $y = \sin(t)$.

The other applet is called Cosine Graph Generator and information technology works in a manner similar to Sine Graph Generator. The web address for this applet is http://gvsu.edu/south/Lz

Properties of the Graphs of the Sine and Cosine Functions

The graphs of $y = \sin(t)$ and $y = \cos(t)$ are called sinusoidal waves and the sine and cosine functions are chosen sinusoidal functions. Both of these detail sinusoidal waves take a period of $two\pi$. The graph over ane period is called a cycle of the graph. As with other functions in our previous study of algebra, some other important property of graphs is their intercepts, in particular, the horizontal intercepts or the points where the graph crosses the horizontal axis. One big difference from algebra is that the sine and cosine functions take infinitely many horizontal intercepts.

In Exercise $\PageIndex{two}$, we used Figure $\PageIndex{2}$ and determined that $-\dfrac{\pi}{2}, \dfrac{\pi}{2}, \dfrac{3\pi}{two}, \dfrac{five\pi}{2}, \dfrac{7\pi}{2}$ are $t$-intercepts on the graph of $y = \cos(t)$. In particular, in the interval $[0, ii\pi]$, the only $t$-intercepts of $y = \cos(t)$ are $t = \dfrac{\pi}{2}$ and $t = \dfrac{3\pi}{2}$.

There are, of grade, other $t$-intercepts, and this is where the menstruation of $2\pi$ is helpful. Nosotros tin can generate any other $t$-intercept of $y = \cos(t)$ by calculation integer multiples of the menstruum $2\pi$ to these two values. For example, if we add $6\pi$ to each of them, we see that $t = \dfrac{thirteen\pi}{2}$ and $t = \dfrac{15\pi}{2}$ are $t$ intercepts of $y = \cos(t)$.

Do $\PageIndex{3}$

Use a graph to determine the $t$-intercepts of $y = \sin(t)$ in the interval $[0, ii\pi]$. Then use the period property of the sine role to determine the $t$-intercepts of $y = \sin(t)$ in the interval $[-2\pi, four\pi]$. Compare this issue to the graph in Figure 2.1. Finally, determine two $t$-intercepts of $y = \sin(t)$ that are not in the interval $[-ii\pi, four\pi]$.

Reply

The graph of $y = \sin(t)$ has $t$-intercepts of $t = 0, t = \pi$, and $t = 2\pi$ in the interval $[0, 2\pi]$.

If we add the period of $2\pi$ to each of these $t$-intercepts and subtract the period of $2\pi$ from each of these $t$-intercepts, we see that the graph of $y = \sin(t)$ has $t$-intercepts of $t = -2\pi, t = -\pi, t = 0, t = \pi, t = two\pi, t = 3\pi$, and $t = four\pi$ in the interval $[-2\pi, 4\pi]$.

We can make up one's mind other $t$-intercepts of $y = \sin(t)$ by repeatedly adding or subtracting the period of $2\pi$. For example, there is a $t$-intercept at:

$t = 3\pi + 2\pi = five\pi$
$t = five\pi + 2\pi = seven\pi$
However, if we look more advisedly at the graph of $y = \sin(t)$, we run across that the $t$-intercepts are spaced $\pi$ units apart. This means that we can say that $t = 0 + 1000\pi$, where $1000$ is some integer, is a $t$-intercept of $y = \sin(t)$.

Activity 2.v (Exploring Graphs of Sine Functions)

Do 1 of the post-obit:

Describe the graphs of $y = \sin(t)$, $y = \dfrac{ane}{2}\sin(t)$ and $y = 2\sin(t)$, $y = -\sin(t)$, and $y = 2\sin(t)$ on the same axes. Make sure your graphing utility is in radian manner and use $-2\pi \leq x \leq 2\pi$ and $-two.five \leq ten \leq ii.five$.
Utilize the Geogebra applet Amplitude of a Sinusoid at the following web accost: http://gvsu.edu/s/LM

The expression for $one thousand(t)$ tin be changed but get out it gear up to $one thousand(t) = \sin(t)$. The slider can be moved to change the value of A and the graph of $y = A\sin(t)$ volition exist drawn. Explore these graphs past irresolute the values of A making sure to use negative values of A every bit well equally positive values of A. (It is possible to alter this to $yard(t) = \cos(t)$ and explore the graphs of $y = A\sin(t)$.

The Aamplitude of Sine and Cosine Functions

The graphs of the functions from Activity 2.5 should have looked similar 1 of the graphs in Figure 2.3. Both graphs are graphs of $y = A\sin(t)$, merely the i on the left is for $A > 0$ and the one on the correct is for $A < 0$. Note that when $A < 0$, $-A > 0$. Another of import characteristic of a sinusoidal moving ridge is the amplitude. The amplitude of each of the graphs in Figure 2.iii is represented by the length of the dashed lines, and we run into that this length is equal to $|A|$.

Definition: aamplitude

The amplitude of a sinusoidal moving ridge is one-one-half the distance between the maximum and minimum functional values.

\[\text{Amplitude} = \dfrac{one}{2}|(\text{ max y-coordinate}) - (\text{min y-coordinate})|\]

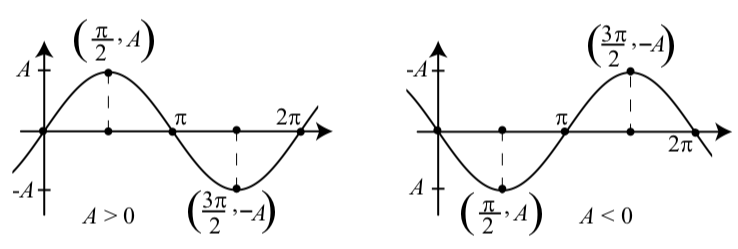
$2.3.png$
Figure $\PageIndex{3}$: Graphs of $y = A\sin(t)$

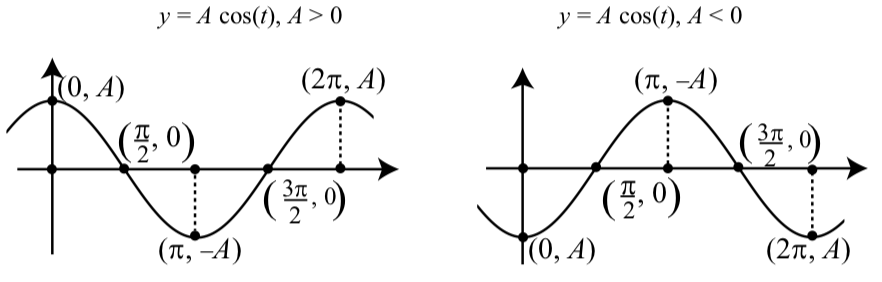
Exercise $\PageIndex{4}$

Draw graphs of $y = A\cos(t)$ for $A > 0$ and for $A < 0$ like to the graphs for $y = A\sin(t)$ in Figure $\PageIndex{3}$.

Respond: $pc 2.6.png$

Using a Graphing Utility

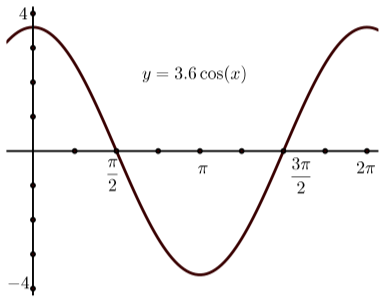
Nosotros often will employ a graphing utility to draw the graph of a sinusoidal function. When doing so, information technology is a practiced idea to use the aamplitude to help set an appropriate viewing window. The basic idea is to have the screen on the graphing utility show slightly more than one period of the sinusoid. For example, if nosotros are trying to draw a graph of $y = 3.vi\cos(t)$, we could use the following viewing window. \[-0.five \leq ten \leq 6.five \space and \space -4 \leq y \leq four.\] If it is possible, set up the $10$-tickmarks to be every $\dfrac{\pi}{iv}$ or $\dfrac{\pi}{2}$ units.

Exercise $\PageIndex{5}$

Apply a graphing utility to describe the graph of $y = 3.6\cos(t)$ using the viewing window stated prior to this progress test.
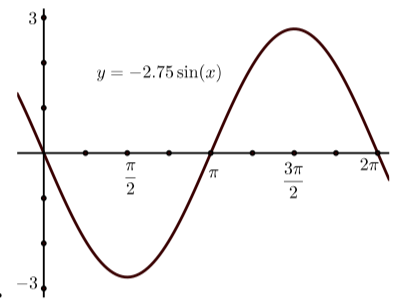
Use a graphing utility to depict the graph of $y = -2.75\sin(t)$.

Reply

one. $2.7.1.png$

2. $2.7.2.png$

Symmetry and the Negative Identities

Examine the graph of $y = \cos(t)$ shown in Figure 2.two. If we focus on that portion of the graph betwixt $-2\pi$ and $2\pi$, we can discover that the left side of the graph is the "mirror epitome" of the correct side of the graph. To encounter this better, use the Geogebra applet Symmetry of the Graph of $y = \cos(t)$ at the following link: http://gvsu.edu/due south/Ot

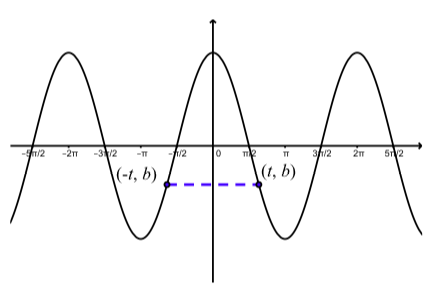
$2.4.png$

Figure $\PageIndex{4}$: Graph Showing Symmetry of $y = \cos(t)$

Figure $\PageIndex{4}$ shows a typical image from this applet. Since the 2nd coordinate of a bespeak on the graph is the value of the role at the first coordinate, this figure (and applet) are indicating that $b = \cos(t)$ and $b = \cos(-t)$. That is, this is illustrating the fact that $\cos(-t) = \cos(t)$. The next action provides an explanation every bit to why this is true.

Activeness 2.eight: Positive and Negative Arcs

For this activity, we will use the Geogebra applet called Drawing a Positive Arc and a Negative Arc on the Unit Circle. A link to this applet is http://gvsu.edu/s/Ol

As the slider for $t$ in the applet is used, an arc of length t volition be drawn in blue and an arc of length $-t$ will exist fatigued in red. In improver, the coordinates of the concluding points of both the arcs $t$ and $-t$ will exist displayed. Study the coordinates of these two points for diverse values of $t$. What practice you detect? Keeping in listen that the coordinates of these points can also be represented every bit \[(\cos(t), \sin(t))\space and \space (\cos(-t), \sin(-t)),\]

what does these seem to signal about the relationship between $\cos(-t)$ and $\cos(t)$? What about the relationship between $\sin(-t)$ and $\sin(t)$?

Effigy $\PageIndex{5}$ shows a typical situation illustrated in Activity 2.8. An arc and information technology corresponding negative arc have been drawn on the unit of measurement circle. What we have seen

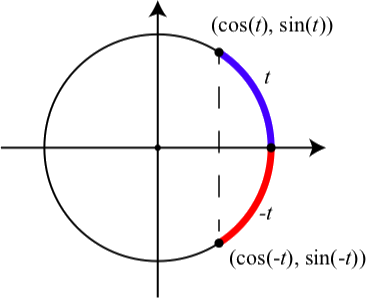
$imageedit_11_5491419173.png$

Figure $\PageIndex{5}$: An Arc and a Negative Arc on the Unit Circle

is that if the terminal betoken of the arc $t$ is $(a, b)$, then past the symmetry of the circle, we encounter that the terminal point of the arc $-t$ is $(a, -b)$. Then the diagram illustrates the following results, which are sometimes called negative arc identities.

Definition: Negative Arc Identities

For every existent number $t$, \[\sin(-t) = -\sin(t) \space \cos(-t) = \cos(t).\]

To further verify the negative arc identities for sine and cosine, use a graphing utility to:

Describe the graph of $y = \cos(-10)$ using $0 \leq x \leq 2\pi$. The graph should be identical to the graph of $y = \cos(x)$.
Describe the graph of $y = \sin(-x)$ using $0 \leq x \leq 2\pi$. The graph should be identical to the graph of $y = \sin(x)$.

These so-chosen negative arc identities requite us a manner to expect at the symmetry of the graphs of the cosine and sine functions. We have already illustrated the symmetry of the cosine part in Figure $\PageIndex{4}$. Because of this, we say the graph of $y = \cos(t)$ is symmetric nearly the y-centrality.

What about symmetry in the graph of the sine part? Effigy $\PageIndex{6}$ illustrates what the negative identity $\sin(-t) = \sin(t)$ implies about the symmetry of $y = \sin(t)$. In this case, we say the graph of $y = \sin(t)$ is symmetric about the origin.

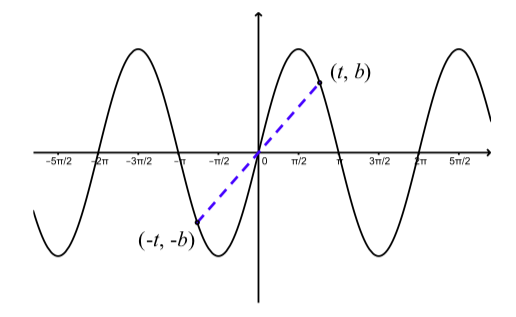
$2.6.png$

Effigy $\PageIndex{6}$: Graph Showing Symmetry of $y = \sin(t)$.

To encounter the symmetry of the graph of the sine function improve, use the Geogebra applet Symmetry of the Graph of $y = \sin(t)$ at the following link: http://gvsu.edu/s/Ou

Summary

In this section, we studied the following of import concepts and ideas:

The important characteristics of sinusoidal functions of the class $y = A\sin(t)$ or $y = A\cos(t)$ shown in Table ii.1.
The information in Table 2.one can seem similar a lot to call up, and in fact, in the next sections, we will get a lot more information about sinusoidal waves. Then instead of trying to think everything in Table 2.1, it is better to retrieve the basic shapes of the graphs as shown in Figure $\PageIndex{7}$ and Effigy $\PageIndex{8}$.

Table two.one: Characteristics of Sinusoidal Functions.
$y = A\sin(t)$		$y = A\cos(t)$
All real numbers	domain	All real numbers
$two\pi$	period	$2\pi$
$\|A\|$	amplitude	$\|A\|$
$(0, 0)$	$y$-intercept	$(0, A)$
$t = 0$ and $t = \pi$	$t$-intercept in $[0, 2\pi)$	$t = \dfrac{\pi}{two}$ and $t = \dfrac{three\pi}{2}$
$\|A\|$	maximum value	$\|A\|$
$-\|A\|$	minimum value	$-\|A\|$
The interval $[-\|A\|, \|A\|]$	range	The interval $[-\|A\|, \|A\|]$
$t = \dfrac{\pi}{ii}$	when $A > 0$, maximum occurs at	$t = 0$
$t = \dfrac{3\pi}{2}$	when $A > 0$, maximum occurs at	$t = \pi$
$t = \dfrac{3\pi}{2}$	when $A < 0$, maximum occurs at	$t = \pi$
$t = \dfrac{\pi}{2}$	when $A < 0$, maximum occurs at	$t = 0$
the origin	symmetry with respect to	the $y$-axis

One manner to remember the location of the tick-marks on the $t$-centrality is to remember the spacing for these tick-marks is one-quarter of a flow and the period is $two\pi$. Then the spacing $\dfrac{two\pi}{4} = \dfrac{\pi}{2}$.

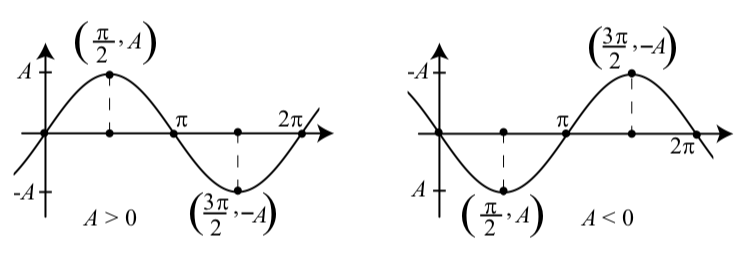
$page100image3422944544$ $page100image3422944832$ $2.7.png$

Figure $\PageIndex{7}$: Graphs of $y = A\sin(t)$.

$2.8.png$

Figure $\PageIndex{viii}$: Graphs of $y = A\cos(t)$.

Supplemental Material – Even and Odd Functions

There is a more than general mathematical context for these types of symmetry, and that has to do with what are chosen fifty-fifty functions and odd functions.

Definitions: fifty-fifty and Odd functions

A office $f$ is an even function if $f(-x) = f(x)$ for all $x$ in the domain of $f$.
A function $f$ is an odd function if $f(-x) = -f(x)$ for all $x$ in the domain of $f$.

And so with these definitions, we can say that the cosine function is an fifty-fifty function and the sine function is an odd function. Why practise nosotros employ these terms? One explanation is that the concepts of even functions and odd functions are used to describe functions $f$ of the form $f(x) = 10^n$ for some positive integer $n$, and the graphs of these functions exhibit different types of symmetry when $n$ is even versus when $n$ is odd.

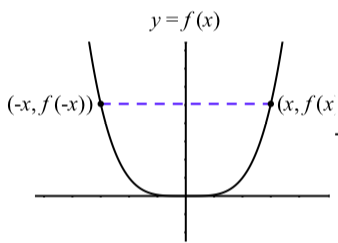
$2.9.png$

Figure $\PageIndex{9}$: $f(ten) = x^n$, n even and $f(-10) = f(10)$

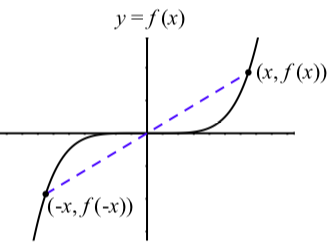
$2.10.png$

Figure $\PageIndex{10}$: $f(x) = ten^n$, $north$ odd and $f(-x) = -f(x)$.

In Effigy $\PageIndex{ix}$, we see that when $n$ is even, $f(-x) = f(x)$ since $(-10)^n = 10^north$. So the graph is symmetric about the $y$-axis. When $n$ is odd equally in Figure $\PageIndex{10}$, $f(-x) = -f(10)$ since $(-x)^n = -x^northward$. So the graph is symmetric about the origin. This is why nosotros apply the term even functions for those functions $f$ for which $f(-x) = f(x)$, and nosotros use the term odd functions for those functions for which $f(-x) = -f(ten)$.

Source: https://math.libretexts.org/Bookshelves/Precalculus/Book:_Trigonometry_%28Sundstrom_and_Schlicker%29/02:_Graphs_of_the_Trigonometric_Functions/2.01:_Graphs_of_the_Cosine_and_Sine_Functions

Posted by: harristuddly.blogspot.com

\(t\)	\(\sin(t)\)	\(\sin(t)\) (approx)
0	0	0
\(\dfrac{\pi}{6}\)	\(\dfrac{one}{2}\)	0.5
\(\dfrac{\pi}{4}\)	\(\dfrac{\sqrt{2}}{ii}\)	0.707
\(\dfrac{\pi}{3}\)	\(\dfrac{\sqrt{3}}{2}\)	0.866
\(\dfrac{\pi}{2}\)	1	ane
\(\dfrac{two\pi}{iii}\)	\(\dfrac{\sqrt{3}}{two}\)	0.866
\(\dfrac{iii\pi}{4}\)	\(\dfrac{\sqrt{2}}{two}\)	0.714
\(\dfrac{five\pi}{vi}\)	\(\dfrac{ane}{2}\)	0.5
\(\pi\)	0	0
\(\dfrac{7\pi}{half-dozen}\)	\(-\dfrac{1}{ii}\)	-0.five
\(\dfrac{5\pi}{iv}\)	\(-\dfrac{\sqrt{ii}}{2}\)	-0.707
\(\dfrac{4\pi}{3}\)	\(-\dfrac{\sqrt{3}}{2}\)	-0.866
\(\dfrac{3\pi}{2}\)	-one	-1
\(\dfrac{five\pi}{3}\)	\(-\dfrac{\sqrt{three}}{2}\)	-0.866
\(\dfrac{vii\pi}{4}\)	\(-\dfrac{\sqrt{2}}{2}\)	-0.707
\(\dfrac{eleven\pi}{vi}\)	\(-\dfrac{one}{ii}\)	-0.v
\(2\pi\)	0	0

\(t\)	\(\cos(t)\)	\(\cos(t)\) (approx)
\(0\)	\(1\)	\(1\)
\(\dfrac{\pi}{half-dozen}\)	\(\dfrac{\sqrt{three}}{2}\)	\(0.866\)
\(\dfrac{\pi}{four}\)	\(\dfrac{\sqrt{2}}{ii}\)	\(0.707\)
\(\dfrac{\pi}{3}\)	\(\dfrac{1}{two}\)	\(0.5\)
\(\dfrac{\pi}{2}\)	\(0\)	\(0\)
\(\dfrac{2\pi}{3}\)	\(-\dfrac{one}{two}\)	\(-0.5\)
\(\dfrac{three\pi}{iv}\)	\(-\dfrac{\sqrt{2}}{2}\)	\(-0.714\)
\(\dfrac{5\pi}{6}\)	\(-\dfrac{\sqrt{three}}{2}\)	\(-0.866\)
\(\pi\)	\(-1\)	\(-1\)
\(\dfrac{7\pi}{half-dozen}\)	\(-\dfrac{\sqrt{3}}{2}\)	\(-0.866\)
\(\dfrac{5\pi}{iv}\)	\(-\dfrac{\sqrt{ii}}{2}\)	\(-0.714\)
\(\dfrac{4\pi}{3}\)	\(-\dfrac{1}{two}\)	\(-0.5\)
\(\dfrac{three\pi}{2}\)	\(0\)	\(-one\)
\(\dfrac{5\pi}{iii}\)	\(\dfrac{i}{2}\)	\(0.5\)
\(\dfrac{7\pi}{iv}\)	\(-\dfrac{\sqrt{2}}{2}\)	\(0.707\)
\(\dfrac{11\pi}{half-dozen}\)	\(\dfrac{\sqrt{3}}{ii}\)	\(-0.866\)
\(2\pi\)	\(one\)	\(i\)

\(y = A\sin(t)\)		\(y = A\cos(t)\)
All real numbers	domain	All real numbers
\(two\pi\)	period	\(2\pi\)
\(\|A\|\)	amplitude	\(\|A\|\)
\((0, 0)\)	\(y\)-intercept	\((0, A)\)
\(t = 0\) and \(t = \pi\)	\(t\)-intercept in \([0, 2\pi)\)	\(t = \dfrac{\pi}{two}\) and \(t = \dfrac{three\pi}{2}\)
\(\|A\|\)	maximum value	\(\|A\|\)
\(-\|A\|\)	minimum value	\(-\|A\|\)
The interval \([-\|A\|, \|A\|]\)	range	The interval \([-\|A\|, \|A\|]\)
\(t = \dfrac{\pi}{ii}\)	when \(A > 0\), maximum occurs at	\(t = 0\)
\(t = \dfrac{3\pi}{2}\)	when \(A > 0\), maximum occurs at	\(t = \pi\)
\(t = \dfrac{3\pi}{2}\)	when \(A < 0\), maximum occurs at	\(t = \pi\)
\(t = \dfrac{\pi}{2}\)	when \(A < 0\), maximum occurs at	\(t = 0\)
the origin	symmetry with respect to	the \(y\)-axis

Which Of The Following Functions Illustrates A Change In Amplitude

ii.one: Graphs of the Cosine and Sine Functions

The Periods of the Sine and Cosine Functions

Properties of the Graphs of the Sine and Cosine Functions

The Aamplitude of Sine and Cosine Functions

Symmetry and the Negative Identities

Summary

Supplemental Material – Even and Odd Functions

0 Response to "Which Of The Following Functions Illustrates A Change In Amplitude"

Post a Comment

Iklan Atas Artikel

Iklan Tengah Artikel 1

Iklan Tengah Artikel 2

Iklan Bawah Artikel